Description
The Curious Hats of Magical Maths Book 2 is the second workbook in the series on Vedic Mathematics. It leads you to unique, enjoyable, and very quick methods of working with numbers. Vedic Mathematics for calculations is carefully described with step-by-step worked examples for you to follow. There are plenty of practice exercises so that you can gain some mastery over the techniques.
This system of Vedic Mathematics was constructed from ancient Vedic sources by Bharati Krsna Tirthaji about a hundred years ago. It is based on sixteen simple rules called sutras, each of which is represented by a "Curious Hat". The sutras briefly encapsulate rules of mental working, principles, or guiding maxims. Through simple practice, you can enjoy mathematics, become adept and quick at mental written calculations, losing any fear of numbers along the way.
This book is a revised, enlarged, and illustrated edition of Vedic Mathematics for Schools Book 2.
The author's understanding of Vedic Mathematics and his qualities as a teacher shine out in these excellent volumes. The simplicity and efficiency of the Vedic system are brought out through the clearly explained examples and there are many exercises and puzzles to stretch the young mind. Easy proofs of all the techniques are given, the topics are well structured and the text is very readable. I must thoroughly recommend these books to students and teachers.
This book is the second edition of Vedic Mathematics for Schools but there are so many changes from the previous edition that it carries a new name. It follows on from The Curious Hats of Magical Maths Book 1. It is aimed at providing enrichment as well as foundation and support material for children in years 8 and 9 (UK) and classes VII and VIII (India), grades 7 and 8 (USA). It concentrates entirely on the Vedic arithmetic and algebraic methods useful for children at these stages in their mathematical education. By the very nature of the subject, however, it can be read and worked through by anyone. This is because most people are unfamiliar with the efficient and delightful methods within Vedic mathematics. So this book does not cover the whole maths syllabus and, being free from the constraints of school curricula, can have a wider appeal.
Since it is a workbook you can write the answers in the spaces provided. There are plenty of examples with step-by-step instructions. You will need to follow the steps carefully before working through each exercise. The answers are in the back and so you can check your work as you go.
One aim of this book is to provide further grounding in the Vedic methods which can then be applied elsewhere in mathematics. Of particular importance is the ability to solve a given problem in different ways because this lays the foundation for learning about strategies.
Vedic Mathematics offers a wonderful approach to solving problems with extremely fast methods for calculating. It's fun and does not take long to learn but you must be prepared to practise. Many of the methods develop further those shown in Book 1 whilst others are introduced for the first time.
By following the system you will also learn about strategies for problem-solving. There is usually more than one way to solve a problem and Vedic Maths is a flexible system that encourages you to find the most efficient method for getting to the answer. For example, if you have to add mentally 239 and 399 you can add 9 and 9, 3 and 9 an 2 and 3, take account of the carry digits and then come up with the answer. That is the conventional method and is quite long. Vedic Maths teaches you to look at the problem in the round, as it were, and say 399 is one less than 400. So I will add 400 to 239 and then subtract 1, making 638. This method will be obvious to most. But the use of a deficiency for multiplication and division is not well known. It does lead to stunningly quick methods for special cases and these are described in this book. Vedic Mathematics deals with both special cases and general cases; and this is worthwhile, particularly where a conventional method is long, slow and cumbersome.
Where does this system come from and why is it called Vedic Maths? During the last century there was a revered spiritual teacher, a Sankaracarya, named Sri Bharati Krishna Tirtha. In his younger days he was a brilliant scholar and was mostly interested in the ancient spiritual teachings of India called Veda. The Veda are texts, thousands of years old, and contain knowledge both spiritual and practical. They contain a philosophy which forms the basis of many Indian religions. Bharati Krishna Tirtha studied these texts, together with associated commentaries and appendices, and hit upon various clues concerning mathematics. Fired by his own love of mathematics he followed the clues and came up with a system based on sixteen rules or sutras. There are also a similar number of sub-rules or sub-sutras. His sutras might not be found in the ancient texts but according to the other meaning of Veda, which has to do with knowledge in the present moment, his methods and sutras can be described as Vedic.
In his introduction he describes the process which ancient seers used for discovering the laws relating to number as true visualisation. It is quite possible that his own experience was similar. His understanding of the word Veda appears to be quite different from the usual version of a collection of ancient texts and relates more to living knowledge. Whatever the case as to the origin of the sutras ''the proof of the pudding is in the eating", as it were. In other words, if it works and is consistent with right reasoning then it should be accepted and if not, then rejected. He encourages us to keep our minds ever open. This is good advice because the methods for such things as multiplication and division are quite different from the conventional long versions. Vedic mathematics leans towards mental methods so that, for example, with practice, you can multiply or divide any numbers in one line. And even when faced with a problem which you solve intuitively, just by looking, this is also included in the system.
It would be a mistake to think that Vedic mathematics provides a complete set of methods different from conventional maths. Yes, it does have many methods which are far slicker, but it also includes conventional methods. For example, when transposing terms from one side of an equation to the other, with a change of sign, this is taught conventionally.
In this case the Vedic system simply points out the sutra which is used for that same process, namely, Transpose and Adjust. So in that sense Vedic maths gives you a different perspective, a different orientation to the mental workings as you solve problems. The sutras describe the patterns of working within your mind.
Sutras
This book uses fifteen of the sutras and sub-sutras and, as with Book 1, many of them are represented by the image of a hat - like a thinking cap! These can be found on the next page.
| Introduction | viii | |
| Prologue | xi | |
| Chapter 1 | The Mighty Vinculum | 1 |
| Chapter 2 | Nikhilam Multiplication | 8 |
| Chapter 3 | Multiplication By Vertically And Crosswise | 18 |
| Chapter 4 | Division Using A Base | 33 |
| Chapter 5 | Squaring | 46 |
| Chapter 6 | Products, Factors, Multiples And Primes | 53 |
| Chapter 7 | Fractions | 62 |
| Chapter 8 | Algebra | 72 |
| Chapter 9 | Casting Out | 82 |
| Chapter 10 | The Working Base | 88 |
| Chapter 11 | Further Fractions | 102 |
| Chapter 12 | Ratio And Proportion | 111 |
| Chapter 13 | Percentages | 119 |
| Chapter 14 | Equatios | 130 |
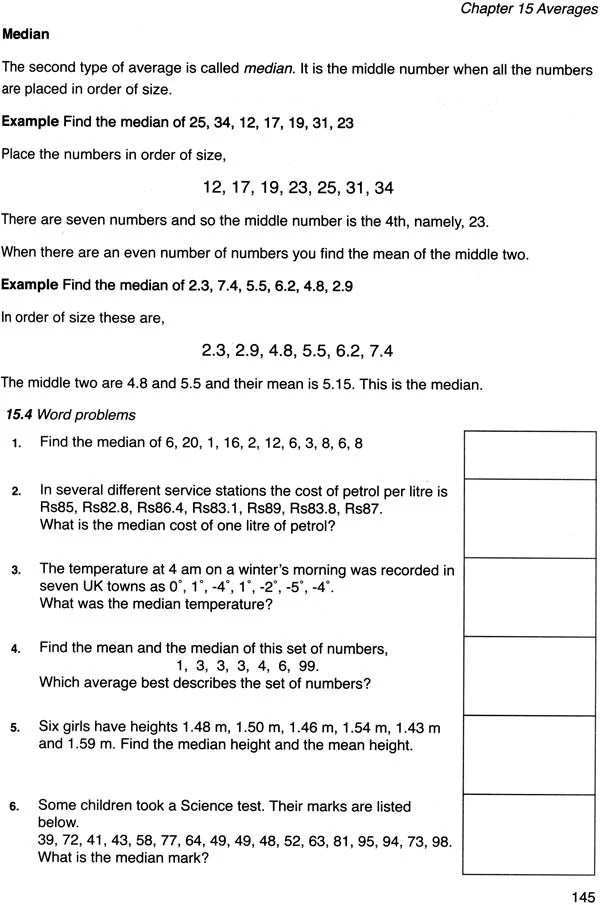
| Chapter 15 | Averages | 142 |
| Chapter 16 | Working With Decimals | 148 |
| Chapter 17 | Straight Division | 154 |
| Chapter 18 | Decimal Fractions | 164 |
| Answers | 176 | |
| Appendix | 202 | |
| Index | 204 |